Why Does The Card Fall?
A classic demo turns out to be a little trickier than I was ready for
I forget exactly how we got on this, but at dinner with SteelyKid and Kate last night1 we wound up talking about the classic air pressure demo of holding water up with paper, which I repeated this morning in our break room using my classic ScienceBlogs coffee mug and an index card:
This is generally presented as a demonstration of air pressure. When the mug with water in it and a card over the top is inverted, the card is subject to three forces: the (tiny) force of gravity on the card itself, the weight of the water pressing down on the card, and an air pressure force pushing up. That air pressure force is substantial— around 10N per square centimeter of the card’s surface— and can easily exceed the weight of the water (a total force of around 3N for the amount of water in that mug), holding the card up. A somewhat crude force diagram would look like this, the green arrows being the gravitational forces, the red the air pressure force:

(Properly speaking there would also be some downward force from the edges of the mug to keep the paper from accelerating upward, but I’m trying to keep this simple…)
It’s a classic “magic trick” science demo, which I did over the sink because somehow SteelyKid hadn’t seen it before. Then Kate asked a question I hadn’t really thought about before: “Why doesn’t it work with air?” That is, the same upward air pressure force should be present when the mug is empty, so why doesn’t that suffice to keep the card in place when the mug is inverted (as shown in the “nothing up my sleeve” portion at the start of the video). I had to think about it a bit, and while I think I figured it out, the fact that I had to stop and think probably indicates that this is worth walking through in a blog post.
So, what’s missing from the explanation? Well, you can see that by thinking about the case where you just have the card all by itself. In that case, there’s an upward air pressure force on the card from the air below it, but also a downward air pressure force from the air above it. Those cancel each other out, so the net force that acts is just the gravitational force on the card itself (at least initially), so it falls.
When you put the card on the inverted mug, that downward air pressure force is acting on the mug, but is counteracted by my hand holding the mug up. So a more complete crude force diagram looks like this:

I’ve added a purple arrow to indicate the support force from my hand, and a downward air pressure at the top of the mug. A not-quite-right-but-sort-of-close way to think about it is that the mug “blocks” the downward force from acting on the paper, leading to the unbalanced situation described above.
But, again, that argument would also seem to hold for the empty mug— it’s blocking the air pressure from above. So why doesn’t the card stay in place when the mug is filled with just air? It should be even easier, because the air doesn’t have to support the weight of the water…
I think the answer has to do with the fact that water is, well, wet. That is, the water makes a bit of a seal at the edge of the card that prevents air from leaking in (not quickly, anyway…). When the mug is empty, there isn’t that same seal, so air from outside can leak in between the edges of the mug and the card (and vice versa). That takes you back to, essentially, the situation where the card is all by itself: the air inside and the air outside are part of the same fluid at the same pressure, so there’s a downward air pressure force that counters the upward air pressure force, leaving only the weight of the card pulling down, so it falls.

At least, I think that’s the explanation of what’s going on, here. I’m sort of at a loss for what would be a conclusive demonstration that this is the answer, though. I think I sorta-kinda have an idea (replace the card with a piece of metal or something and make a seal around the edge using a bit of oil or something that isn’t sticky enough to hold up the metal on its own, but will if you block the air pressure at the top), but I’m not confident. This may be a reasonable excuse to dick around in the lab a bit, though, which would probably be good for me…
Addendum: A couple of people have reminded me that the air in the bubble that’s visible in the mug must at least start out at atmospheric pressure, which would complicate this picture— while I drew it with an air bubble, I talked about it as if there’s no air above the card.
The answer to this must be that the bubble expands slightly (either through the card flexing or some water leaking out), lowering the pressure inside. That gives a pressure imbalance again— the air in the bubble pushes out with less pressure than the air outside, and the difference between the resulting forces supports the water.
The one thing that’s slightly hard to square with that is that this works substantially better with stiffer card stock, which would flex less, than regular paper. I’m moderately certain I remember doing it with a metal sheet, as well. But I’d guess that’s a question of the quality of the seal— lighter paper that stretches more is more likely to leak and allow extra air in.
So, there’s a little bit of physics to end your week. If you’d like to see whether I actually go dick around with oil and metal plates to test this, here’s a button:
And if you have any suggestions or corrections, the comments will be open:
We also fed The Pip, lest you think we’re Bad People. But he’s a 13-year-old boy, so he inhaled his dinner in about 45 seconds and then retired to the couch to watch YouTube videos about the NBA draft on his phone.


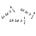

I have never seen this so I went and tried it and it is quite surprising to me that it works! My card was bending a little and there was actually a visible bead of water about 1/4 inch along two of the sides between the small square plastic container and the paper index card. Surface tension of the water seems to be what is keeping the water from flowing out sideways.
Just to put some math to it.... Say your mug has a diameter of 75 mm, which gives a cross sectional area of A = 4400 mm^2 = 0.0044 m^2, and a height h = 100 mm. That's a volume of V = A*h = 440 mL. Let's say it's f = 2/3 full of water, for a water height of hw = 66.7 mm, and a water volume Vw = fV = 293 mL. That means there's an air height of (1-f)h = 33.3 mm, and an (initial) air volume of Va1 = (1-f)V = 147 mL of air inside. The water has a mass of m=Vw*ρ, where ρ=1 g/mL = 1000 kg/m^3, so m 293 g. We'll assume the paper and air are both negligible mass. So, to hold that in the cup, you need a force of F = m * g, where g = 10 m/s^2, F = 2.93 N.
As the water and card drop a little, the gas in the mug will expand, lowering the pressure from atmospheric to some internal pressure Pi. But we still have atmospheric pressure acting on the bottom of the card, Po = 100 kN/m^2. That creates a pressure difference, Po - Pi, between the inside and outside the mug. The pressure difference, acting on the area of the mug cross section, creates the force. (Note, the pressure acting up on the card outside of the mug cross section is balanced by the pressure acting down on the other side of the card outside the mug cross section.) We have F = (Po - Pi)A = 2.93 N. So, Pi = Po - F/A, F/A = 665 N/m^2, and Pi = 99.33 kN/m^2.
If we assume there's no energy transfer to/from the gas as it expands (the system is adiabatic), which seems reasonable, then PV^γ is constant, where P is pressure, V is volume, and γ is the heat capacity ratio, which is 1.4 for air. We can use that to find how much the volume of air inside the cup needs to expand to create that pressure. With an expanded air volume Va2, we have
Po*Va1^γ = Pi*Va2^γ
Va2/Va1 = (Pi/Po)^(-1/γ) = (99.33/100)^(-1/1.4) = 1.0048
So, the volume expansion of the air to create the force necessary to hold the water up is pretty small, about 0.5%. Since the coffee mug is a cylinder, that translates directly to the change in height of the air. So we're talking about going from 33.3 mm of air to 33.5 mm. And that 0.2 mm change in height means that's how much of the water is exposed to air at atmospheric pressure, trying to push into the mug. I won't (read: am not smart enough to) compute how small that gap has to be for the surface tension of the water to prevent "swallowing" an air bubble, but, intuitively, that's small enough.
Algebraically, after substitutions,
Va2/Va1 = ((Po - F/A)/Po)^(-1/γ)
ha2/ha1 = (1 - f*h*ρ*g/Po)^(-1/γ)
The change in air height is
Δh = (1-f*h) * ((1 - f*h*ρ*g/Po)^(-1/γ) - 1)
Note, that's entirely independent of the cross section of the mug (assuming it's constant vertically, or at least the same at the mouth and at the air gap). For a 100 mm tall mug, that's going to range between 0 to 0.2 mm back to 0 of height change in the air as you fill the mug from empty* to full, which, again, certainly seems small enough. But as the height of the mug increases, the change in air height grows - if you're drinking one of those yard-long margaritas in New Orleans to celebrate the Super Bowl, that change in height will range between 0 to 19 mm back to 0, and surely that's big enough to leak.
*Also note, in my earlier comment, I had asked, what's the dividing line for the amount of water necessary to hold the card up? This analysis doesn't find one, but that's because we assumed the card mass was negligible. As the amount of water drops, this is no longer a good assumption.