The academic term at Union ended a couple of weeks ago, which always leaves me feeling drained and a little burned out, a feeling that is aggravated by a very great deal of stupid and angry commentary in a variety of formats. This accounts for my longer-than-usual silence last week; I was trying my best to stay away from dumb fights.
In a similar spirit, I decided to spend some time puttering around doing things that have a more positive influence on my general mood. One of these picks up on something I did in my Spring term class, which was to have students in our upper-level lab course do a video analysis lab. They needed to identify a situation involving some interesting physics, make a video of it, and then analyze that video using the Tracker Video Analysis program to generate numbers.
This was kind of a mixed bag as far as the actual student output (in the form of lab reports and class presentations) goes, but a number of them identified problems that were kind of cool and fun. So I’ve decided to take a crack at doing a few of these myself: making videos, cranking them into Tracker, and looking at the output. I don’t have a formal outlet for these— I’m not doing it for a grade in a class, and I don’t know that any of what I’m likely to do would merit publication even in a pedagogical journal— but I’ll write them up here, because I need #content for the blog.
The first of these is something I put a student on to based on a video demo I remembered seeing somewhere: using a magnetic pendulum and a copper block to look at eddy currents. The key physics here is that if you have a magnet near a chunk of non-magnetic metal (copper, aluminum, etc.) it doesn’t experience a force while it’s standing still. When you start to move the magnet, though, the changing magnetic field inside the metal cause the electrons to experience a force that pushes them in circles, and these little loops of current generate their own magnetic fields, which act back on the magnet.
The net effect of this is that a moving magnet near a block of copper feels a force that opposes its motion. This is useful for things like magnetic braking, because the brake and the thing being slowed do not need to be in physical contact with one another, and thus experience less wear and tear than traditional disk brakes and the like. This is also related to the physics behind the popular demo of levitating a small magnet above the surface of a superconductor.
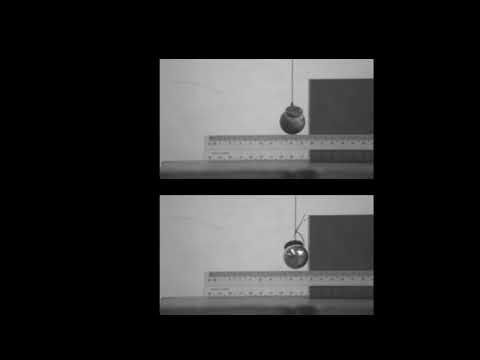
In the context of the kinds of everyday moving objects you can do video analysis with, this shows up as a force slowing the motion of a magnet near a chunk of copper. One way to do this in a fairly controlled way is to make the magnet into a pendulum and swing it so it collides with the block, in which case we see a stark difference between the behavior of a non-magnetic ball bearing and a magnetic ball of the same size and mass:
The non-magnetic ball bounces several times, losing a bit of energy each time and gradually settling in to a position where it’s at rest next to the block. (The video doesn’t quite get to that point, because I only have so much patience…) The magnetic ball experiences a force that slows it down as it comes in, and again as it starts to bounce back, leading to strikingly different behavior: while it does bounce back, the distance it moves is a small fraction of that for the magnetic ball, and then it slowly settles in to the surface.
This behavior can be quantified using Tracker. The trickiest part of this is getting it to automatically mark the position of the ball— doing it by hand for the several hundred frames of video is tedious and error-prone— but a little bit of fiddling got the clips you see above, which were high-contrast enough for the “Autotrack” feature to do a decent job measuring the position. The one issue is that there’s so much data for it to track that the program tends to run out of memory and crash. The screenshot below is actually from a different video clip than the one I used in the video, for that reason:
This shows the basic look of Tracker at some intermediate point in the motion: you can see the red dots indicating the position it deduced for a bunch of previous frames, and the graphs showing the overall motion. I’m a little picky about graphs, so I copied the tabular data at the lower right into a spreadsheet to give me a little more freedom to tweak things around, and got the following (for the actual clips in the YouTube video linked above):
This is a nice qualitative demonstration of the effect, showing that the bounce-back distance for the magnetic ball is about a quarter that of the plain steel one, and that there isn’t a second bounce, just a slow settling in.
But, of course, this is meant to be physics, so we’d like something sort of quantitative about the force arising from the eddy currents. So, what can we say about that? Well, that’s the nice thing about using a pendulum for this: at the turnaround point, we know the mass isn’t moving, and the only forces acting should be from the string and gravity, which allows you to make a simple prediction of what the net force acting on the mass ought to be. We can also deduce the force that’s actually acting from the acceleration determined from the video, and compare the two.
If I take the first bounce of the non-magnetic ball and fit a parabola to the peak of it, I get an acceleration of about 0.8 m/s/s, where the geometry would predict around 0.6 m/s/s. That’s actually pretty good given that it’s a pretty crude fit and I’ve made some simplifying approximations because I’m trying to bang this out quickly. For the magnetic ball, the geometry would predict an acceleration of around 0.15 m/s/s, but the peak there is notable asymmetric. Splitting it in half gives an acceleration of 0.34 m/s/s on the way out (the early side of the peak) and 0.08 m/s/s on the way back in. That’s exactly the sort of thing we ought to expect: on the way out, it feels a force holding it back, which adds to the gravitational force pulling it back toward the center, and on the way back in it feels a force pushing out, reducing the acceleration from gravity.
Of course, you’d like to be able to do something a little more sophisticated than that, but it’s complicated somewhat by the fact that the position data is inherently noisy, which makes a mess when you start looking at derivatives. When I did the first tests by hand, the accelerations determined by Tracker were just garbage.
The auto-track feature is shockingly good, though, leading to this (for the clips from the screenshot, not the ones in the video):
This is the horizontal acceleration as determined by Tracker for the fraction of a second just before and just after the ball hits the block. The acceleration due to gravity ought to be zero here, and you can see that it is for the non-magnetic ball (give or take), other than a brief gap right around zero where it’s huge and negative (cropped out of this plot) as the ball reverses direction within about three frames of video. The magnetic ball, though, shows a rapidly increasing negative acceleration as the ball comes in to collide with the block, then a rapidly decreasing positive acceleration after the collision. This is exactly what we’d expect from the eddy current effect, and shows that you need to be within a centimeter or so for the induced force to be really substantial.
(I totally did not expect this part to work, by the way…)
There are a couple of refinements to this that I may or may not try out in the next week or so— doing some more careful measurements of the geometry to hopefully get the quantitative results a little better, and maybe getting some clips of both balls bouncing off a non-conducting surface (a cinder block, say), in which case there shouldn’t be much difference in the behavior. On the other hand, this is basically sufficient for the primary purpose I have for it, which is to be a class demo and discussion question in future sections of intro E&M.
And that’s the kind of thing I get up to when I’m in need of mood elevation…
So, yeah, there’s a bunch of physics stuff that only like eight people will read in any detail. But, you know, I had fun. If you want to see whether I revisit this, or just move on to some other video analysis thing, here’s a button:
And if you’d like to point out that I’m an enormous dork for enjoying this, believe me I already know, but the comments will be open:





Unintuitive in that the force slows down the ball whether it is approaching the copper or moving away from it (if I am interpreting that right).
It occurs to me that I didn't actually put the specs of the video in the post, so I'll leave them as a comment: The video was shot at 240 frames per second using a Chronos camera that a former colleague bought some years back. It's monochrome, which is why everything looks grey.